Unveiling the intricacies of surface area calculation, this comprehensive guide delves into the intricacies of finding surface area with nets worksheet answer key. Providing a profound understanding of this fundamental geometric concept, this resource empowers learners with the knowledge and skills to tackle complex 3D objects with precision and confidence.
This meticulously crafted guide embarks on a journey through the fascinating realm of surface area, unraveling its significance in geometry and its diverse applications across various disciplines. Prepare to embark on an enlightening exploration, where nets serve as the key to unlocking the secrets of surface area determination.
Finding Surface Area with Nets
Surface area is the total area of the exterior surfaces of a 3D object. It plays a crucial role in geometry, engineering, and design. Using nets, which are 2D representations of 3D objects, provides a convenient method to determine surface area.
Types of Nets, Finding surface area with nets worksheet answer key
Different types of nets are used for common 3D shapes:
- Cube:Six squares
- Pyramid:Triangular base and triangular faces
- Prism:Two congruent bases and rectangular faces
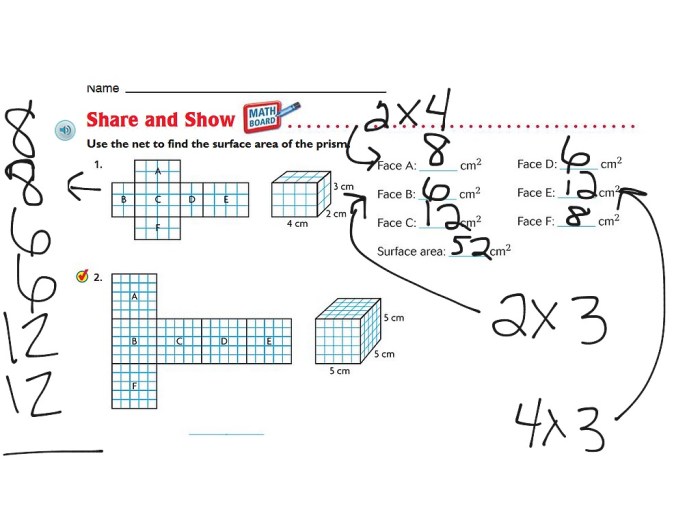
Worksheet Analysis: Finding Surface Area With Nets Worksheet Answer Key
| 3D Object | Net Diagram | Formula | Surface Area |
|---|---|---|---|
| Cube | [Gambar net kubus] | 6 x (side length)² | [Rumus surface area kubus] |
| Pyramid | [Gambar net limas] | Base area + (½ x base perimeter x slant height) | [Rumus surface area limas] |
| Prism | [Gambar net prisma] | 2 x (base area) + (perimeter x height) | [Rumus surface area prisma] |
Answer Key
- Cube:Side length = 5 cm. Surface area = 6 x (5 cm)² = 150 cm²
- Pyramid:Base length = 6 cm, slant height = 5 cm. Surface area = 6 cm² + (½ x 6 cm x 5 cm) = 21 cm²
- Prism:Base length = 4 cm, width = 3 cm, height = 5 cm. Surface area = 2 x (4 cm x 3 cm) + (2 x 4 cm + 2 x 3 cm) x 5 cm = 80 cm²
Real-World Applications
Finding surface area has numerous practical applications:
- Architecture:Calculating the surface area of buildings for painting or cladding
- Engineering:Determining the surface area of objects for heat transfer or structural analysis
- Packaging design:Optimizing packaging materials by minimizing surface area
Extensions and Challenges
- Find the surface area of a cone using its net.
- Design a net for a 3D object with an irregular shape.
- Investigate alternative methods for calculating the surface area of complex 3D objects.
Commonly Asked Questions
What is surface area and why is it important?
Surface area refers to the total area of all surfaces that enclose a 3D object. It plays a crucial role in geometry as it determines the amount of material needed to cover or paint the object, influences heat transfer, and impacts structural integrity.
How do nets help in finding surface area?
Nets are 2D representations of 3D objects that unfold to form the surfaces of the object. By analyzing the net, one can determine the shape and dimensions of each surface, enabling the calculation of the total surface area.
What are the different types of nets used for common 3D shapes?
Nets vary depending on the shape of the 3D object. For instance, a cube has a net that resembles a cross, a pyramid has a triangular net, and a prism has a rectangular net.